IN DEFENSE OF ALEXANDER, by Nikos A. Salingaros
HALI: The International Magazine of Antique Carpet and Textile Art, volume 78 (1995), pages 67-69.
Nikos A. Salingaros, a professor of mathematics at the University of Texas at San Antonio, is both an artist and a scientist. As a HALI reader who has followed the work of Christopher Alexander for the last fifteen years, he believes that Ian Bennett's recent review of A Foreshadowing of 21st Century Art failed to address Alexander's central contribution -- the provision of "a set of tools to judge beauty". In this riposte he argues for the value of Alexander's analysis, and offers an example of the manner in which it can be applied to any art object, in this instance, a standard Tekke Turkoman ensi.
Ian Bennett, in his review of Christopher Alexander's book A Foreshadowing of 21st Century Art (HALI 74, pp. 87-95) concentrates mainly on his many technical disagreements with Alexander; while these concerns are important to oriental carpet enthusiasts, they drew attention away from other very interesting topics. The review in question missed, in my opinion, Alexander's central contribution: a set of tools to judge beauty. These can enhance one's appreciation of art and its relation to life in general. For this reason, his book should be read by a wide variety of people interested in what makes things beautiful and alive.
Professor Alexander connects geometrical patterns with aspects of physical reality. Although hinted at many times in the past by other authors, never to my knowledge has an explicit result appeared. Alexander provides a solid connection that links design and the visual environment on the one hand, with beauty and living forms on the other. The art historian Professor Oleg Grabar posed the central problem of recognition in art: "What makes us recognize or identify a bird, a fish, writing, or a leaf, when what we see does not bear any real resemblance to what we call it?" [The Mediation of Ornament, Princeton University Press, 1992]. Art historians have discussed this question for many years without coming up with a precise answer. Alexander has obtained a solution following his long study of oriental rugs.
Although it might at first appear to be a separate issue, this question also relates to how one can quantify beauty. A friend, who is a Zen Master, oriental art scholar, and past rug dealer, said immediately that one should leave such questions alone, and not try to analyze what quantitative features make one fall in love with a beautiful carpet. "It just happens; and you know when it does -- it is somehow improper to ask why it happens and to try to analyze it".
Alexander has probed into this 'forbidden' topic because he is an architect, and wants to make beautiful buildings. He has, not surprisingly, discovered that in order to make beautiful buildings, you have to know how to make beautiful objects of whatever size. Further, he has discovered rules for making beautiful things from studying the patterns on very old carpets. These rules are universal, and they help to make anything beautiful, from a doll to an apartment complex.
This result is entirely independent from its source, which is the old carpets. Alexander outlines the rules for making beautiful things in the first quarter of his book. The basic building block of any design he calls a 'center'. This is a perceptible region of coherent space. For example, a dot in the center of a page holds the page together; also a circle or square in a page (although not necessarily in the middle of a pattern). Adjoining and overlapping centers can either reinforce each other, or detract from, each other, and they do not need to be symmetric. Centers also interact at a distance. The best patterns or designs are simply those which can be decomposed into the maximum number of mutually reinforcing centers.
Alexander illustrates this process by reference to the carpet designs, using sketches that isolate the various centers. For example, the 'small pattern Holbein', has hundreds of different centers, all densely packed. Even a superficially simple design, if it evokes profound feeling, turns out to contain an enormous number of mutually reinforcing centers. Of course, one does not ordinarily decompose a carpet into its 'field of centers' in order to judge how attractive it is. It happens, however, that in side-by-side comparisons of two carpets (or any other similar objects), the one with the more intense field of centers is invariably the one which is most attractive to most people.
Alexander also introduces another novelty that is very useful in practice. He compares any two objects, and asks which of the two most resembles one's own inner self -- one's character or one's soul. Usually, there is a clear choice, and the one chosen is that which contains the most intense field of centers. This 'mirror of the self' test makes use of basic instincts that humans already have about the field of centers. Showing two similar rugs to novices and asking them to choose according to the 'mirror of the self' test, they usually choose the most valuable one. Amazingly, they may prefer the brighter colors or thicker wool pile of the other, but are nevertheless forced to choose the one that most resembles their conception of self.
There is a further and inescapable conclusion. If one constructs inanimate objects with a very intense field of centers, they begin to resemble living things. This result is surprising. If one applies the field of centers analysis to any living object, animal or plant, then one is overwhelmed by its intensity. Therefore, the rules for creating beautiful things lead to inanimate objects that have the subconscious feeling of living things, without necessarily looking like any living thing at all. And here is the answer to Oleg Grabar's question on representation via abstract forms. An abstract drawing by a master (who intuitively creates a dense field of centers) can convey more of the essential sense of a living thing than a photograph.
Mr. Bennett implies that Alexander's theory of centers and internal unity is somehow already contained in European art historical writing. I cannot agree. Contemporary art and architecture contain almost none of the beauty that is found in old carpets: this is what drives the antiques market, as well as subscriptions to HALI. People just don't know how to make beautiful objects anymore, having absorbed during their education rules that guarantee ugliness. In the very same issue of HALI, there is a picture of the incredibly ugly new exposition hall of the Österreichisches Museum für angewandte Kunst in Vienna. This was created according to the aesthetic canons of today, and will probably even win an architectural prize. In order to prevent such monstrosities, and to perpetuate the beauty that we (the readers of HALI) see in carpets, then Alexander's conclusions should be given their proper due.
Finally, I don't know where Mr. Bennett got the idea that Alexander's rules fail to apply to carpets outside the present collection -- that is simply not true. The aesthetic rules apply to all things, and most certainly to all types of carpets. Alexander's personal preference for 'very early Turkish' carpets (because he finds in them the most intense field of centers) should not detract from the universal applicability of his rules. These rules illustrate unequivocally why carpets can indeed be great works of art.
ANALYSIS OF A TEKKE ENSI

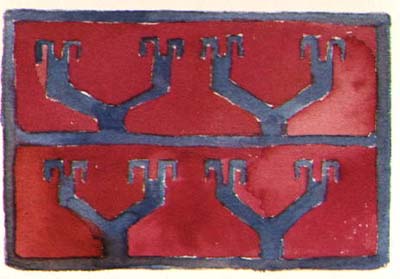
Tekke ensi, 19th century. Author's collection
The following analysis illustrates Alexander's theory of centers and internal unity in a 'standard' type of carpet, touching only on some of the most obvious points. While the rug in question is from Professor Salingaros' own collection, the specific analysis could apply to any ensi.
First and foremost, a hierarchy of scales exist in the ensi. If I
measure the bases of clearly defined rectangles, I find dimensions
that decrease roughly by factors of three. Starting from the width of
the rug, which is 126cm, I have: 61, 18, 8, 3, 1, 0.4, down to 0.2cm
for the line with a 1-knot width -- eight levels of scale in all.
There is some element of design -- a center -- that focuses one's
interest on each of these scales.
1. Six rectangular centers (below, a-f) are drawn to the same scale. The dimension of the base and the number of appearances for each are: a. 59.0 cm (x2), b. 17.5cm (x14), c. 9.2cm (x10), d. 8.5cm (x65), e. 6.4cm (x75), f. 6.0cm (x34).

Figure 1a
The Ensi contains centers that can be used as units. Some rectangular centers are shown in (1 a-f). They are all drawn the same size for comparison, and I have given the dimension of their base and their multiplicity, which is the number of times they appear in the carpet. Each center has an internal complexity and could be further decomposed into smaller centers. For example, (1b) and (1c) are subcenters of (1a).

Figures 1b and 1c
Now there are two distinct ways in which the centers in (1) interact to produce internal cohesion in the carpet. First, each one of them repeats, as indicated by its multiplicity. A repeating pattern ties that space together. This happens not only for adjacent centers, but any two similar centers will link at a distance and establish a connection.


Figures 1d and 1e
The second way to tie the space together is through similarity at different scales, a more subtle process. The rectangular centers in (1) have different dimensions but the same proportion, and they also have many structural similarities. These similarities connect all the different centers across scales, thus linking the different scales. This leads to internal cohesion. One could go on, noting how lines continue across adjacent but distinct centers and tie them on a larger scale, and so on.

Figure 1f
Lastly, each element of the rug, and the rug itself, has a very wide border, of comparable size to what it surrounds. This is obvious for the big rectangles, yet is also true for all the smaller design elements. Unity is achieved because what is surrounding is connected to what is being surrounded. The object and its surroundings together form a larger center.
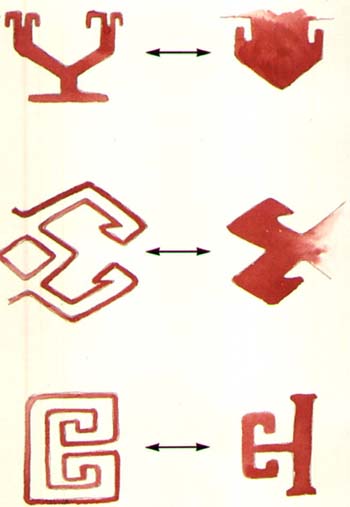
Figure 2. the duality of an object and its background in three different pairs. In each, two opposite figures complement and give life to each other: one cannot tell which is the figure and which is the background.
The wide boundary concept is demonstrated in another way by the duality between an object and its surroundings. 'Duality' here denotes the complementarity of opposites that together form a balanced union -- male/female, yin/yang for example. In a good design there is no left-over space; no distinction between the pattern and its background. Paired examples are sketched in (2). Our analysis implies that the essence of a carpet lies in the degree to which it is internally cohesive.
The Alexander type of analysis can be used to judge whether one rug is better than another similar or dissimilar rug. A quantitative measure of beauty is really useful when it can be applied in comparing objects. For example, comparing other ensis to my own, we see some that are better, others worse, and this can indeed be explained by the comparative density of internal patterns.